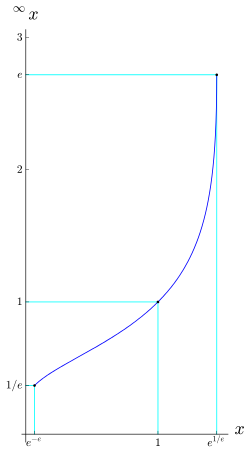
lim
n
→
∞
x
n
{\displaystyle \lim _{n\rightarrow \infty }x^{\frac {n}{}}}
(
e
−
1
)
e
≤
x
≤
e
e
−
1
)
{\displaystyle (e^{-1})^{e}\leq x\leq e^{e^{-1}})}
Tetraatio (”iteroitu potenssiinkorotus”) on matemaattinen laskutoimitus, jossa lukua korotetaan itsensä suuruiseen potenssiin useita kertoja peräkkäin:
Yhteenlasku
a
+
n
=
a
+
1
+
1
+
⋯
+
1
⏟
n
{\displaystyle a+n=a+\underbrace {1+1+\cdots +1} _{n}}
a :han lisätään "1" n kertaa.kertolasku
a
×
n
=
a
+
a
+
⋯
+
a
⏟
n
{\displaystyle a\times n=\underbrace {a+a+\cdots +a} _{n}}
a lisätään itseensä n kertaa.potenssi
a
n
=
a
×
a
×
⋯
×
a
⏟
n
{\displaystyle a^{n}=\underbrace {a\times a\times \cdots \times a} _{n}}
a kerrotaan itsellään n kertaa.Tetraatio
n
a
=
a
a
⋅
⋅
a
⏟
n
{\displaystyle {^{n}a}=\underbrace {a^{a^{\cdot ^{\cdot ^{a}}}}} _{n}}
a korotetaan potenssiin n kertaa.Tetraatiolla merkitään erittäin suuria potenssiinkorotuksia:
4
2
=
2
2
2
2
=
2
[
2
(
2
2
)
]
=
2
(
2
4
)
=
2
16
=
65
,
536
{\displaystyle \,\!\ ^{4}2=2^{2^{2^{2}}}=2^{\left[2^{\left(2^{2}\right)}\right]}=2^{\left(2^{4}\right)}=2^{16}=65,\!536}
On huomattava, ettei potenssiinkorotus ole liitännäinen :
2
2
2
2
≠
[
(
2
2
)
2
]
2
=
2
2
⋅
2
⋅
2
=
256
{\displaystyle \,\!2^{2^{2^{2}}}\neq \left[{\left(2^{2}\right)}^{2}\right]^{2}=2^{2\cdot 2\cdot 2}=256}
x
{\displaystyle x}
2
x
{\displaystyle {}^{2}x}
3
x
{\displaystyle {}^{3}x}
4
x
{\displaystyle {}^{4}x}
1
1
1
1
2
4
16
65,536
3
27
7,625,597,484,987
exp
10
3
(
1.09902
)
{\displaystyle \exp _{10}^{3}(1.09902)}
4
256
exp
10
2
(
2.18788
)
{\displaystyle \exp _{10}^{2}(2.18788)}
exp
10
3
(
2.18726
)
{\displaystyle \exp _{10}^{3}(2.18726)}
5
3,125
exp
10
2
(
3.33931
)
{\displaystyle \exp _{10}^{2}(3.33931)}
exp
10
3
(
3.33928
)
{\displaystyle \exp _{10}^{3}(3.33928)}
6
46,656
exp
10
2
(
4.55997
)
{\displaystyle \exp _{10}^{2}(4.55997)}
exp
10
3
(
4.55997
)
{\displaystyle \exp _{10}^{3}(4.55997)}
7
823,543
exp
10
2
(
5.84259
)
{\displaystyle \exp _{10}^{2}(5.84259)}
exp
10
3
(
5.84259
)
{\displaystyle \exp _{10}^{3}(5.84259)}
8
16,777,216
exp
10
2
(
7.18045
)
{\displaystyle \exp _{10}^{2}(7.18045)}
exp
10
3
(
7.18045
)
{\displaystyle \exp _{10}^{3}(7.18045)}
9
387,420,489
exp
10
2
(
8.56784
)
{\displaystyle \exp _{10}^{2}(8.56784)}
exp
10
3
(
8.56784
)
{\displaystyle \exp _{10}^{3}(8.56784)}
10
10,000,000,000
exp
10
3
(
1
)
{\displaystyle \exp _{10}^{3}(1)}
exp
10
4
(
1
)
{\displaystyle \exp _{10}^{4}(1)}






![{\displaystyle \,\!\ ^{4}2=2^{2^{2^{2}}}=2^{\left[2^{\left(2^{2}\right)}\right]}=2^{\left(2^{4}\right)}=2^{16}=65,\!536}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a62ba9ff84c6ef312eebc36abaa75fbf1d39edf9)
![{\displaystyle \,\!2^{2^{2^{2}}}\neq \left[{\left(2^{2}\right)}^{2}\right]^{2}=2^{2\cdot 2\cdot 2}=256}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bdd629e459e22a4af2885ea2872879604bdb2f4e)


















